Work and Energy - Class 9 Science Notes
- Curator OYI
- Feb 22, 2023
- 5 min read
Updated: Apr 2, 2023
The energy required for the life process comes from food. Activities like playing, singing, reading, writing, thinking, jumping, cycling and running require energy.
Work
Work done on an object is defined as the product of the magnitude of the force acting on the body and the displacement in the direction of the force.
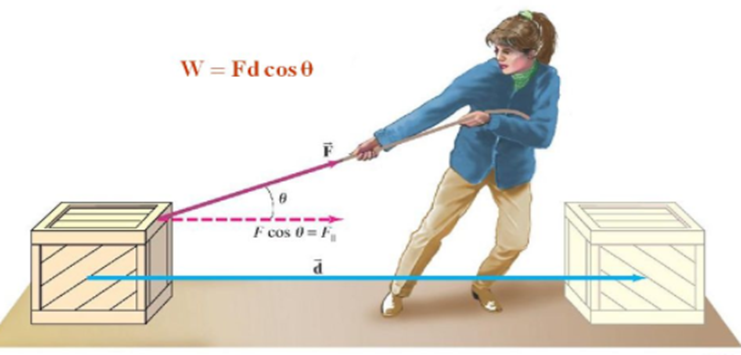
The force component F cos θ gives the component of force along the direction that the body is displaced.
Cos θ is the angle between the force vector and displacement vector.
If a force acting on a body causes no displacement, the work done is 0. For example, pushing a wall.
Work is said to be done when:
1. When a moving object comes to rest.
2. An object at rest starts moving.
3. Velocity of an object changes.
4. Shape of an object changes.
Scientific conception of work
Work is done when force produces motion in body
Work is said to be done when a force is applied on a body and body moves under the influence of the force.
Work done by a Constant Force
Work is done in moving a body is equal to the product of force and the displacement of the body in the direction of the force.

Work done = Force × Displacement
W = F.s.
The SI unit of force is Newton. The other unit is joule (J)
1 joule (J) = 1 Newton Meter (Nm)
Work has only magnitude and no direction.
The amount of work done depends on the following factors:
(i) Magnitude of Force: Greater the force, greater is the amount of work and vice-versa.
(ii) Direction of Force: Greater the displacement, greater is the amount of work and vice-versa.
Work done by force can be positive, negative or zero.

a. Work done is positive when force acts in the direction of motion of the body.
Example: A child pulls toy car with a string horizontally on the ground.

b. Work done is negative when force acts in opposite direction of motion of the body.
Example: Climbing the stairs
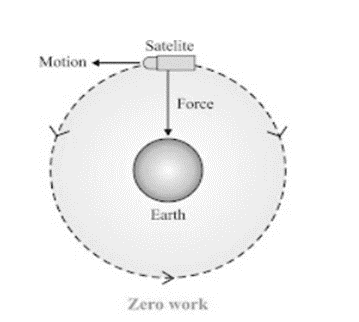
c. Work done is zero when force acts at right angle to the direction of motion.
Example: The moon moves around the earth in circular path. Here force of gravitation acts on the moon at right angle to the direction of motion of the moon. So, the work done is zero.
Energy
The capacity to do work is called Energy.
i. The Sun is the biggest source of energy.
ii. Most of the energy sources are derived from the sun.
iii. Some energy is received from the nucleus of the atoms, interior of the earth and the tides.
The amount of energy possessed by the body is equal to the amount of work it can be done.
Working body losses energy, body on which work is done gains energy.
Energy is scalar quantity.
The S.I. unit of energy is joule (J).
Forms of Energy
i. Kinetic energy Mechanical energy: sum of kinetic and potential energy.
ii. Potential energy Energy possessed by a body on account of its motion
or position is called mechanical energy.
iii. Heat energy
iv. Chemical energy
v. Electrical energy
vi. Light energy
Kinetic Energy
The energy of a body due to its motion is called kinetic energy.

Examples of kinetic energy:
A speeding car
A rolling stone
A flying aircraft
Flowing water
A running athlete
Derivation of formula of kinetic energy:
If an object of mass ‘m’ moving with uniform velocity ‘u’, it is displaced through a distance ‘s’. constant force ‘f’ acts on it in the direction of displacement. Its velocity changes from ‘u’ to ‘v’.
Then acceleration is ‘a’.
Work done, W = F × s ………(i)
F = ma …………(ii)
According to third law of motion, relationship between u, v, s and a is as follows:
v2 – u2 = 2as
s = (v2 – u2)/2a ………. (iii)
Now putting the value of f and s from equation (ii) and (iii) in equation (i), we get,
W = ma × (v2 – u2)/2a
W = m (v2 – u2)/2
If the object is starting from its stationary position, that is, u = 0, then,
W = 1/2 mv2
It is clear that the work done is equal to the change in the kinetic energy of an object. Thus, the kinetic energy possessed by an object of mass m and moving with uniform velocity v is,
Ek = 1/2 mv2
Hence, the kinetic energy is directly proportional to the mass and square of the velocity.
Potential Energy
The energy possessed by a body due to its change in position or shape is called the potential energy.
Examples:
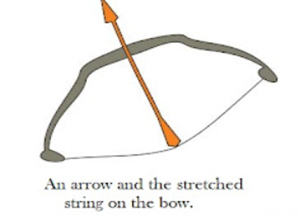
(i) Water kept in dam: It can rotate turbine to generate electricity dure to its position above the ground.
(ii) Bent string of Bow: Potential energy due to change of its shape (deformation) released in the form of kinetic energy while shooting the arrow.
(iii) A rock sitting on the edge of the cliff.

Factor that affects the potential energy:
i. Mass of the body:
P.E α m
More the mass, greater the potential energy and vice-versa.
ii. Height above the ground:
P.E. α h (does not depend on the path it follows)
Greater the height, greater the potential energy and vice-versa.
iii. Change in the shape:
Greater the stretching, twisting or bending, more the potential energy.
Potential energy of an object at a height

If an object of mass m is raised to height h from the ground, the gravitational pull of the earth (m×g) acts in downward direction.
To lift the body, we have to do work against the force of gravity.
Thus, work done is,
Work done = Force × displacement
W = mg × h
W = mgh
This work is stored in the body in the form of potential energy (Gravitational potential energy: it is defined as the work done in raising the object from the ground to a point against the gravity)
Ep = mgh
Where, g = acceleration due to gravity.
The work done by gravity depends on the difference in vertical heights of the initial and final positions of the object and not on the path along which the object is moved.
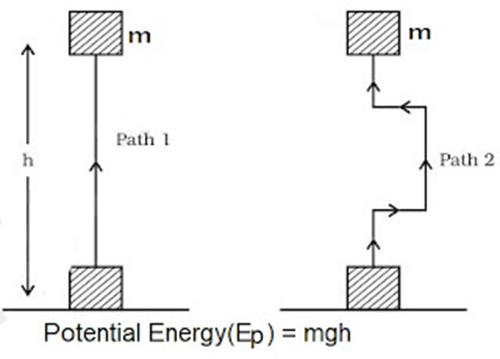
In the above figure a block is raised from position A to B by taking different paths , but in both situation the work done (or potential energy) is mgh.
Transformation of Energy
The change of one form of energy to another form of energy is called transformation of energy.
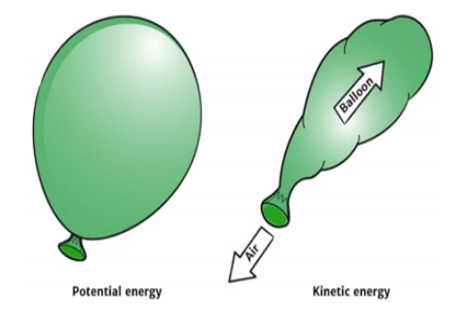
Examples:
(i) An inflated balloon has potential energy in the stretched rubber, while in deflated balloon the air is released in the form of kinetic energy.
(ii) At hydroelectric power house the potential energy of water is converted into kinetic energy and then into electrical energy.
(iii) Plants use solar energy to make chemical energy in the food by the process of photosynthesis.
Law of Conservation of Energy
The law states that, ‘Energy can only be converted from one form to another; it can neither be created nor be destroyed. The total energy remains the same’.
Although some amount of energy gets wasted during process but the total energy before and after the transformation remains same.
The law is valid in all situations and for all kinds of transformations.
Conservation of energy during free fall of body:
- A ball of mass ‘m’ at a height ‘h’ has potential energy, mgh.
- As ball falls downwards, height ‘h’ decrease, so the potential energy also decreases.
- Kinetic energy at ‘h’ is zero but it increases as it approaches ground.
- The sum of the potential energy and kinetic energy of the ball remains same at every point during its fall.
Potential energy + Kinetic energy = Constant
mgh + 1/2 mv2 = Constant
The sum of kinetic energy and potential energy of an object is its total mechanical energy.
Rate of Doing Work (Power)
Power is defined as the rate of doing work or the rate of transfer of energy. Or
Power is the rate of energy consumption.
Power = Work done
Time taken
P = W/t
Unit of Power
Power is one Watt when one Joule work is done in one second. S.I. unit of power is Watt.
1 W = 1 joule/second
Relation between kilo watt hour and joule:
1 kWh = 1 kW × 1 h
= 1000 W × 3600 s
= 3600000 J
1 kWh = 3.6 × 106 J
